Return to Assignments Fractional functions and graphs
Assignment 7
Given the function:
Find:
the vertical asymptote;
the horizontal asymptote;
the intersection point with the 
-as if it exists;
the intersection point with the 
-as if it exists.
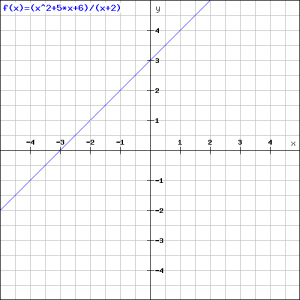
Based on these results sketch the result in the figure.
Solution
The line 
seems to be an asymptote. However, when we substitute this value in the formula we get 
. That the numerator yields 
for means that the numerator contains the factor 
. Indeed, the numerator can be factorized: 
. Now the function can be rewritten:
if 
.
We do not need to do complicated calculations any more. There also no asymptotes. The graph of the function is equal to the graph of 
, excluded the point 
. The graph approaches 
if 
approaches 
The graph in the figure is valid, exluded the point .
