Return to Assignments Fractional functions and graphs
Assignment 3
Given the function:
![]()
Find:
the vertical asymptote;
the horizontal asymptote;
the intersection point with the ![]() -as if it exists;
-as if it exists;
the intersection point with the ![]() -as if it exists.
-as if it exists.
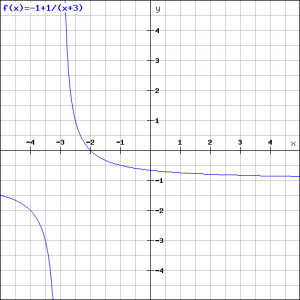
Based on these results sketch the result in the figure.
Solution
The vertical asymptote is ![]() . We find the horizontal asymptote when
. We find the horizontal asymptote when ![]() or
or ![]() and that is
and that is ![]() , because the second term approaches
, because the second term approaches ![]() and thus
and thus ![]() approaches
approaches ![]() . The intersection point with the
. The intersection point with the ![]() -axis is
-axis is ![]() . This result can be obtained by substituting
. This result can be obtained by substituting ![]() in the function. The intersection point with the
in the function. The intersection point with the ![]() -as is obtained by substituting
-as is obtained by substituting ![]() and solving the following equation:
and solving the following equation:
![]()
The solution is: ![]()
See the graph in the following figure: