Return to Assignments Optimizing
Assignment 8
Verify whether the function:
has a maximum or a minimum.
Solution
Naturally we have .
Candidates for a maximum or a minimum can be found by making the first derivative equal to and solve the resulting equation. We find the derivative using the quotient rule:
A fraction equals if its numerator equals
and thus we have to solve:
and thus:
or
In order to investigate whether a point is a maximum or a minimum we could use the second derivative. However this requires a lot of calculations. It is easier to consider the sign of the first derivative. The denominator is always positive, we only have to look at the numerator. This contains a quadratic function of which the graph is an 'opens up' parabola. Around the left intersection point
the first derivative goes from + via
to - and thus there is a maximum for
. For
the reverse holds and thus we have a minimum for this point.
The extremes are thus (maximum) and
(minimum).
This result seems strange: a minimum that is greater than a maximum but it is not. Look at the graph below. Notice that the line is an asymptote.
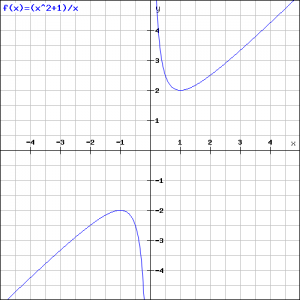
There is also another approach. Before differentiating the function we first simplify it by dividing both numerator and denominator by .
This function is easier to handle and it immediately shows the existence of the asymptote .
Return to Assignments Optimizing

