Return to Assignments Exponential functions and graphs
Assignment 10
For which value(s) of do the graphs of the functions:
have only one point in common.
Solution
In order to calculate the intersection points of and
we have to solve the following equation:
Substituting:
in the equation we get:
This quadratic equation has two coinciding solutions if the discriminant , so if:
or
If we get
, i.e.
and this does not give a solution. Thus the value of
for which both graphs have just one common point is
.
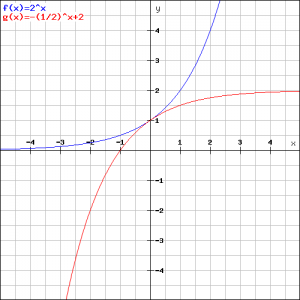
Verify that the common point is , see the figure.