Return to Assignments Absolute value |x|
Assignment 6
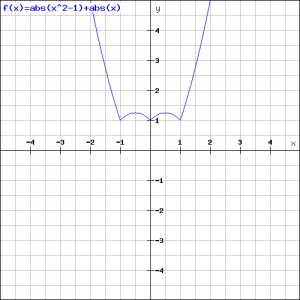
Draw the graph of
Solution
We apply the definition of the absolute value to both terms.
if
, so if
or
(see also assignment 4). Call this interval A.
if
. Call this interval B.
if
. Call this interval C.
if
. Call this interval D.
To draw the graph we distinguish the following intervals.
, this is the union of the intervals A and D, briefly AD.
, this is the union of the intervals B and D, briefly BD.
, this is the union of the intervals B and C, briefly BC.
, this is the union of the intervals A and C, briefly AC.
For AD we have:
For BD we have:
For BC we have:
For AC we have:
The resulting graph is built up by portions of four different parabolas. The first part intercepts and has a minimum for
. Calculate the same characteristics for the other parabolas and explain them based on the chart. Note that the graph fits the given function.
Return to Assignments Absolute value |x|